hdu5528(積性函式+尤拉函式)
題意:設(題目已把f(6)的表給出),
,給定n(n<=1e9),求g(n)
最主要的是求f(m),考慮到模數大於0的情況比較多,所以考慮考慮求ij mod n==0的情況。。
然後其實從題目給的表中看出對一個a來說,他0的個數和gcd(a,n)有關,其實也很容易證明,對一個數a來說,取完gcd部分,其餘部分都是多餘的,所以只要考慮gcd的部分產生的影響就行,然後就差n/gcd這些因子,即只要b取的是n/gcd的倍數,那麼就可以使ab為n的倍數,那麼這些b共有n/(n/gcd)=gcd個,所以對每個a,他產生ab餘數為0的個數為gcd(a,n),即
然後
為積性函式,可以通過素因子分解求出
設,由於h(n)符合狄利克雷卷積形式,故h(n)也為積性函式
而
因此h(n)也可通過素因子分解求出。。
複雜度為O(Tsqrt(n))
然後又被卡常qaq
分解的時候可以列舉sqrt(n)以內的素因子分解,實測會快10倍左右。。
/** * ┏┓ ┏┓ * ┏┛┗━━━━━━━┛┗━━━┓ * ┃ ┃ * ┃ ━ ┃ * ┃ > < ┃ * ┃ ┃ * ┃... ⌒ ... ┃ * ┃ ┃ * ┗━┓ ┏━┛ * ┃ ┃ Code is far away from bug with the animal protecting * ┃ ┃ 神獸保佑,程式碼無bug * ┃ ┃ * ┃ ┃ * ┃ ┃ * ┃ ┃ * ┃ ┗━━━┓ * ┃ ┣┓ * ┃ ┏┛ * ┗┓┓┏━┳┓┏┛ * ┃┫┫ ┃┫┫ * ┗┻┛ ┗┻┛ */ #include<cstdio> #include<cstring> #include<algorithm> #include<iostream> #include<queue> #include<cmath> #include<map> #include<stack> #include<set> #include<bitset> #define inc(i,l,r) for(int i=l;i<=r;i++) #define dec(i,l,r) for(int i=l;i>=r;i--) #define link(x) for(edge *j=h[x];j;j=j->next) #define mem(a) memset(a,0,sizeof(a)) #define ll unsigned long long #define eps 1e-8 #define succ(x) (1LL<<(x)) #define lowbit(x) (x&(-x)) #define sqr(x) ((x)*(x)) #define NM 200015 #define nm 200005 #define pi 3.1415926535897931 using namespace std; const ll inf=1e9+7; ll read(){ ll x=0,f=1;char ch=getchar(); while(!isdigit(ch)){if(ch=='-')f=-1;ch=getchar();} while(isdigit(ch))x=x*10+ch-'0',ch=getchar(); return f*x; } int n,tot,prime[NM],m; ll _ans,ans; bool v[NM]; void init(){ m=40000; inc(i,2,m){ if(!v[i])prime[++tot]=i; inc(j,1,tot){ if(i*prime[j]>m)break; v[i*prime[j]]++; if(i%prime[j]==0)break; } } } int main(){ init(); int _=read();while(_--){ scanf("%d",&n);_ans=ans=1; for(int i=1;sqr(prime[i])<=n&&i<=tot;i++)if(n%prime[i]==0){ int t=1;ll s=1,k=1; while(n%prime[i]==0)n/=prime[i],t++,s*=prime[i],k+=sqr(s); _ans*=t*s;ans*=k; } if(n>1)_ans*=2*n,ans*=1ll*n*n+1; ans-=_ans; printf("%llu\n",ans); } return 0; }
Count a * b
Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 262144/262144 K (Java/Others)
Total Submission(s): 1367 Accepted Submission(s): 464
Problem Description
Marry likes to count the number of ways to choose two non-negative integers a
Let's denote f(m) as the number of ways to choose two non-negative integers a and b less than m to make a×b mod m≠0.
She has calculated a lot of f(m) for different m, and now she is interested in another function g(n)=∑m|nf(m). For example, g(6)=f(1)+f(2)+f(3)+f(6)=0+1+4+21=26. She needs you to double check the answer.
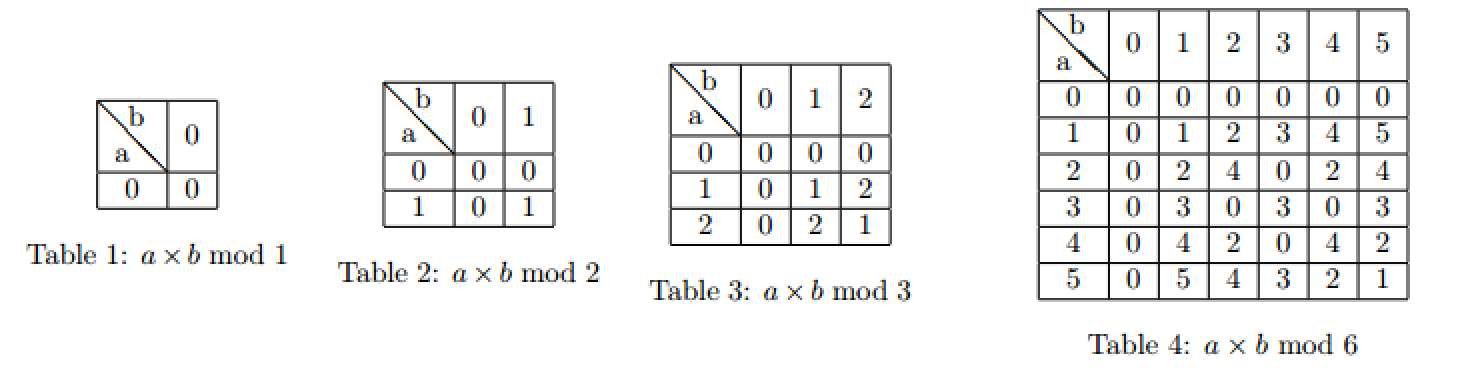
Give you n. Your task is to find g(n) modulo 264.
Input
The first line contains an integer T indicating the total number of test cases. Each test case is a line with a positive integer n.
1≤T≤20000
1≤n≤109
Output
For each test case, print one integer s, representing g(n) modulo 264.
Sample Input
2 6 514
Sample Output
26 328194
Source
2015ACM/ICPC亞洲區長春站-重現賽(感謝東北師大)
Recommend
hujie | We have carefully selected several similar problems for you: 6447 6446 6445 6444 6443
