Fourier Transforms and the Buddhist Doctrine of No-Self

Joseph Fourier discovered the extraordinary fact that some functions can be written as an infinte sum of harmonics. The Fourier Transform is an extension of this initial discovery, and is a highly used technique in engineering, physics and many other disciplines. The crux of it is this:

You can decompose a signal (a function of time) into its component frequencies

When two waves interact with each other, they don’t simply pass through one another, nor do they collide like particulate matter; they interfere. The height of the peak of a wave is its amplitude, and when waves interfere the result is a new waveform with a larger, smaller or similar amplitude. The more waves involved, the more complex the resultant waveform.

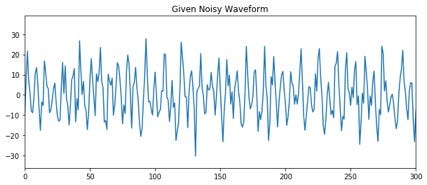
The above waveform is a messy combination of a couple of waves with various frequencies. Using the mathematical tool of a Fourier Transform, we can discover how many waves make up our original waveform and what each of their frequencies are.

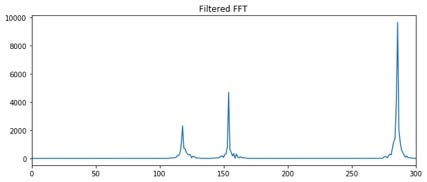
The three peaks show that our waveform is composed of three different waves with the corresponding frequencies on the graph. What we have just done is truly astonishing. Consider a fully baked cake. We have been able to deduce both what

Moving to Buddhism, one of the most powerful teachings in its arsenal is its description of the Three Marks of Existence.

Annica — Impermanance, Dukkha — Suffering, Annata — No Self

I want to speak to annata, the Doctrine of No Self. This teaching is not a negation of the existence of the phenomenal world, but rather, an argument against the intrinsic and independent existence of objects. The contents of the world are all interdependently originated. Nothing contains an essence and nothing exists in and of itself. Objects, including ourselves, are like the waveform above. They can be decomposed and analyzed with respect to thier components. It is important to note that these components themselves are not intrinsic features of reality, they too are interdependently arisen from the web of the cosmos. The ‘decomposition’ of our notion of Self has been known for millenia in Buddhism. Our egoic sense of Self, our I, is a combination of five ‘frequencies’, or in Buddhist terminology, Five Skandhas or Aggregates.

Form — Feeling — Perception — Mental Formations — Consciousness

If we try to look for our Self, all we will find are the Skandhas. I posit that the analogous technique to the Fourier Transform is Buddhist Vipassana meditation. It can be used to enable a meditator to engage in a systematic search for his sense of self. A prerequisite to this technique is Anapanasati, the ability to concentrate and focus the mind. Using this, one can carefully breakdown and examine where exactly our apparent sense of ‘I’ is located. Upon deep introspection, it is found to be illusory, and exists only as an emergent property of the Five Skandhas. We reify the dynamical relationship of the Skandhas and ascribe permanance to them, calling them a Self.

The Fourier Transform breaks things down and enables us to analyze and alter their components — you can change the predominance of a certain frequency in a waveform, or get rid of it all together. Similarly, by practicing Vipassana, you become aware of your personality’s constructed nature: especially the Mental Formations (in Sanskrit called Sankharas). These encompass the way in which you react to certain sensations/feelings (called vedana in Sanskrit). Our personalities are largely built upon our habitual reactions to situations. At a primal level, we tend to react with craving to pleasant sensations and aversion to unpleasant sensations. With increasingly sharp awareness and equanimity, it is possible to alter the way in which we react. We can diminish certain patterns or strengthen others. While this is an advanced goal, at the very least, we can strive to simply become aware of the existence of the patterns. This in itself increases our agency, leading to a more harmonious and conscious mode of existence.