A Young Student’s Approach to Polygon Areas

Sharing the work of Sameer Sharma, age 16.

Consider the sequence of regular polygons of side length 1. Actually, consider the sequence of their areas. The plot of these values seems to resemble a quadratic curve.

Given the emphasis we place on quadratics and their graphs in the upper middle school and high school curricula, it would not at all be unreasonable for a young student to presume that this data is following a quadratic formula. Afterall, in the universe of Algebra I graphing what other curved graphs are there?

What a delight it was for me to receive a particular email from a young lad from India six months ago. Sameer was playing with these areas, plotted the data, saw the shape of the curve being traced, but decided there was no reason to believe the data was actually quadratic in nature. He had been watching and reading my

But the point of his email was to share with me a quadratic expression he claimed to be a very good approximation to the data nonetheless. He added that my course on quadratics had led him to this formula.

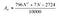
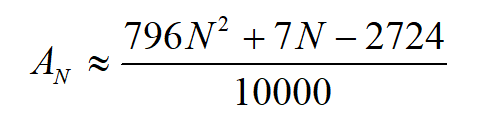
I was intrigued. How did those notes lead him to a quadratic approximation? And is he right? Does his quadratic produce good approximations for the data?

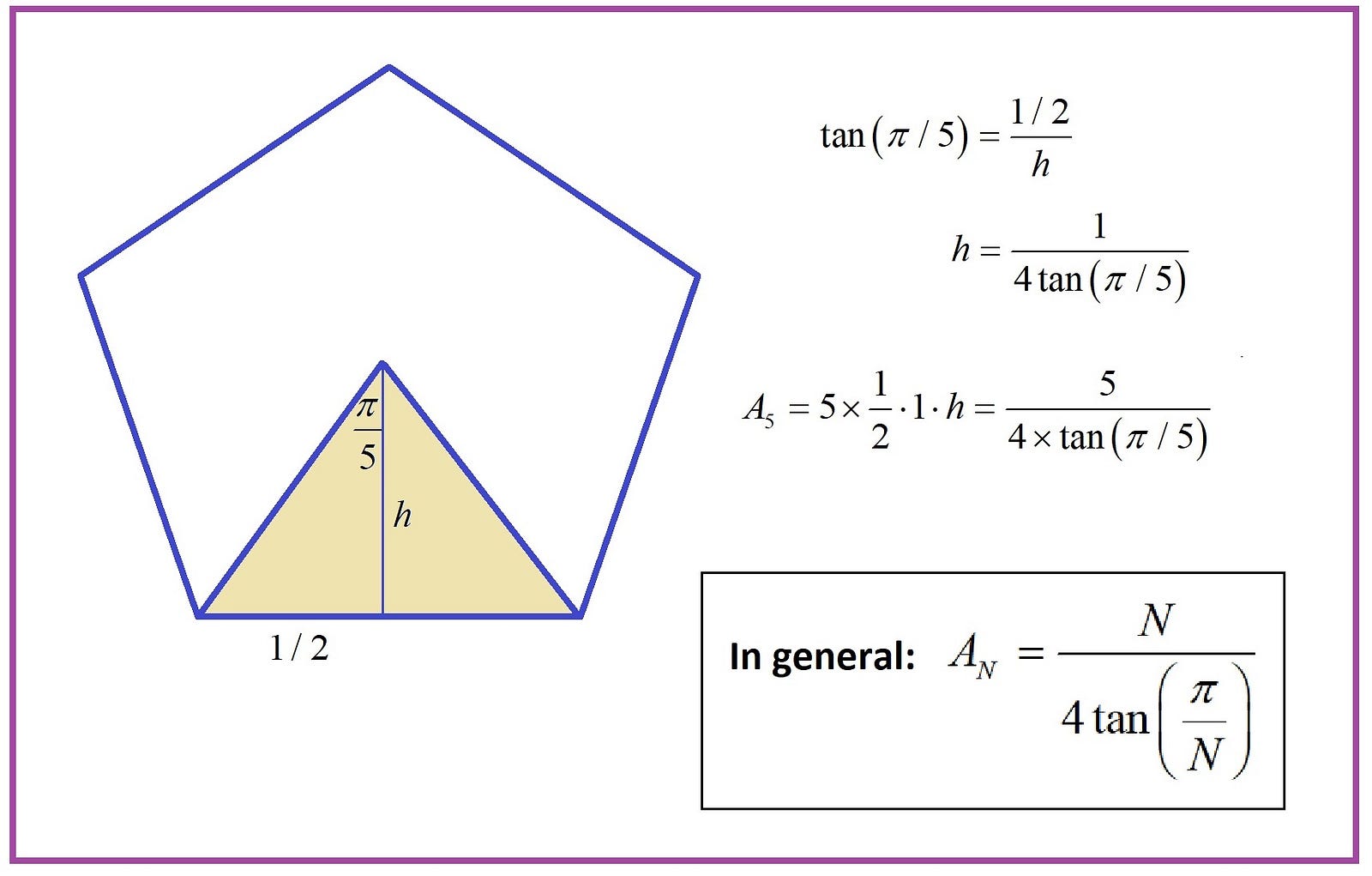
An exercise in trigonometry gives the formula for the area of each regular N-gon. (The data is certainly not quadratic!)

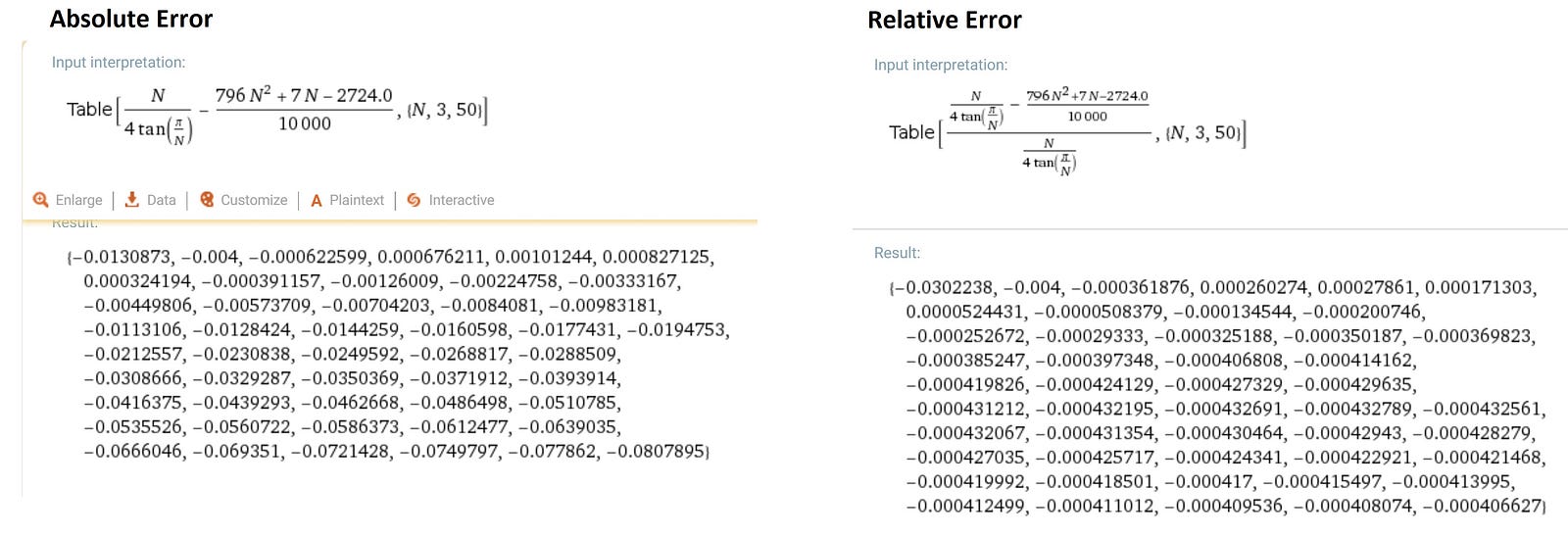
And a quick run through with Wolfram Alpha gives the absolute errors and the relative errors between Sameer’s quadratic formula and the trigonometric formula. Looks good!

Then I wondered: How would I obtain a quadratic approximation? My brain went straight to advanced calculus. I used the Laurent expansion of cot(x)! (See endnote too.)

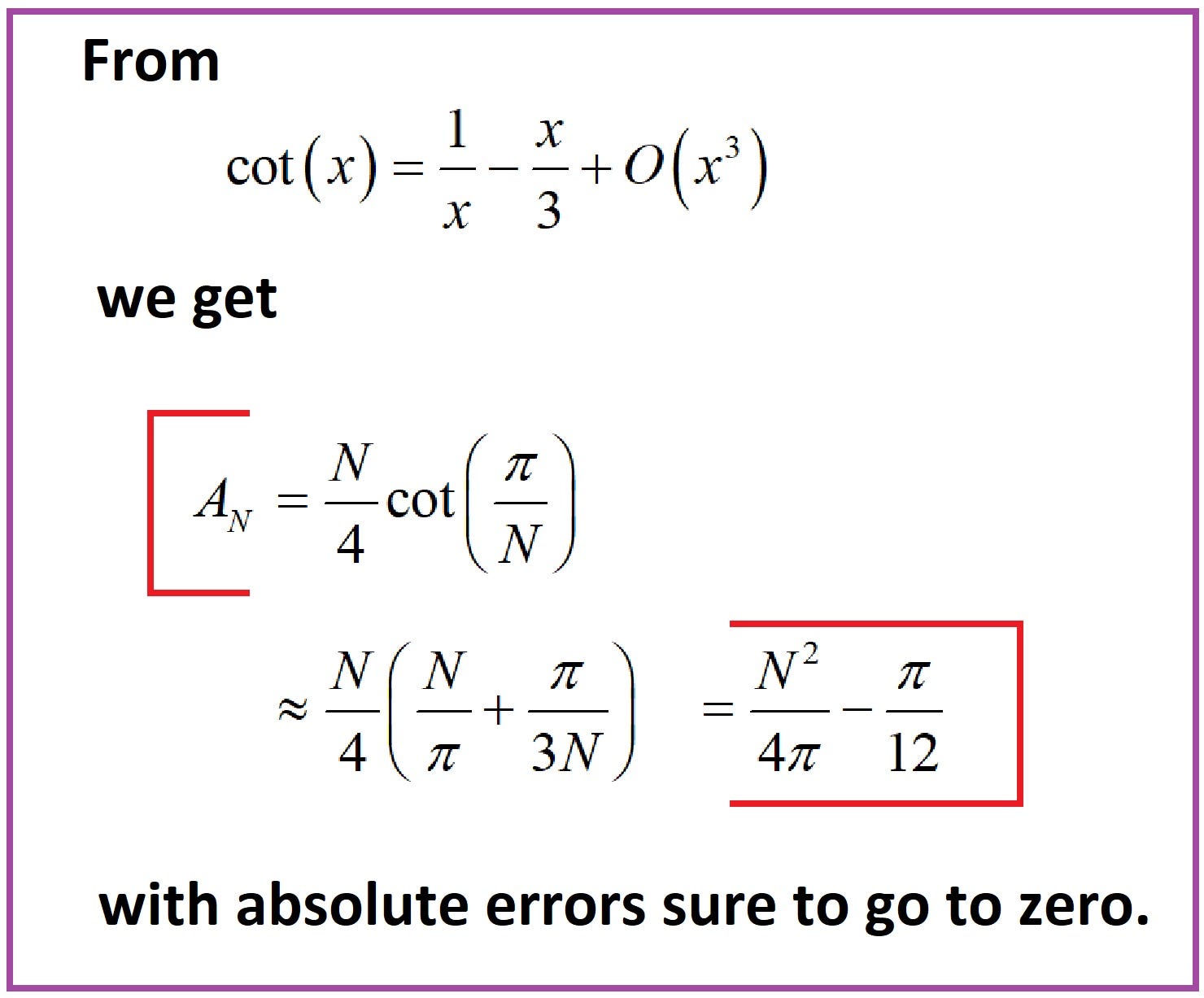
Approximating the coefficients as four-digit decimals, this formula reads A = 0.0796* N² — 0.2618. The leading coefficient is the same as Sameer’s!

Okay … so what did Sameer do?

It took some coaxing to find out as Sameer felt his approach wasn’t “true” mathematics. That was not at all the case.

Sameer had indeed been watching my course on quadratics and learned that if a sequence of numbers has differences that increase at a constant rate, then that sequence is given by a quadratic expression. Moreover, it is possible to write down what that expression is.

Sameer looked at the first few area terms, did not see constant double differences (the data is not quadratic!), but decided to start by pretending they were. With trial and error he massaged and tweaked his numbers to obtain a quadratic expression that seemed to work well. Here’s how he describes his process.

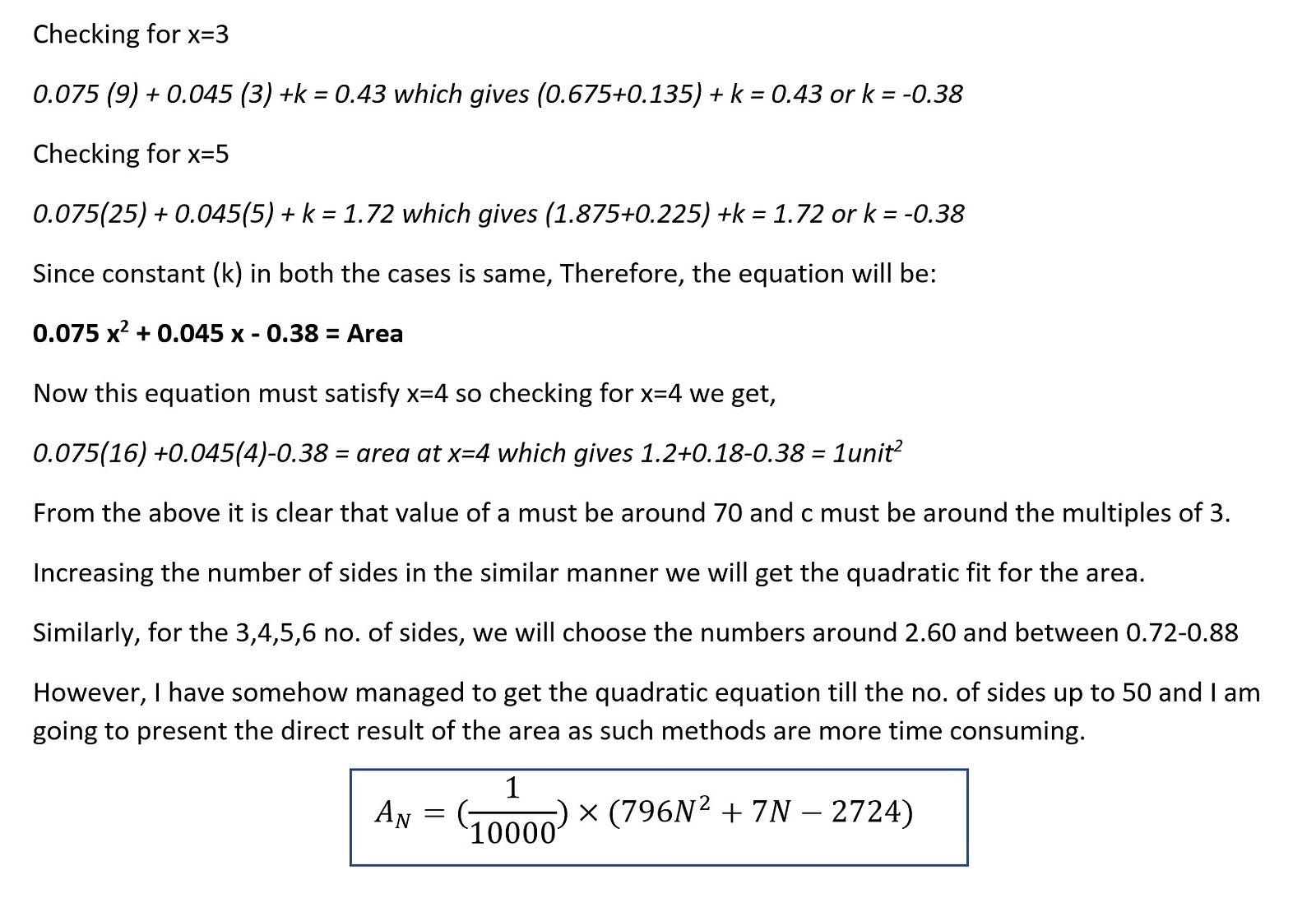
Later, after an exchange of emails back and forth, I shared my “calculus” formula with Sameer and he plotted this diagram of their absolute errors.

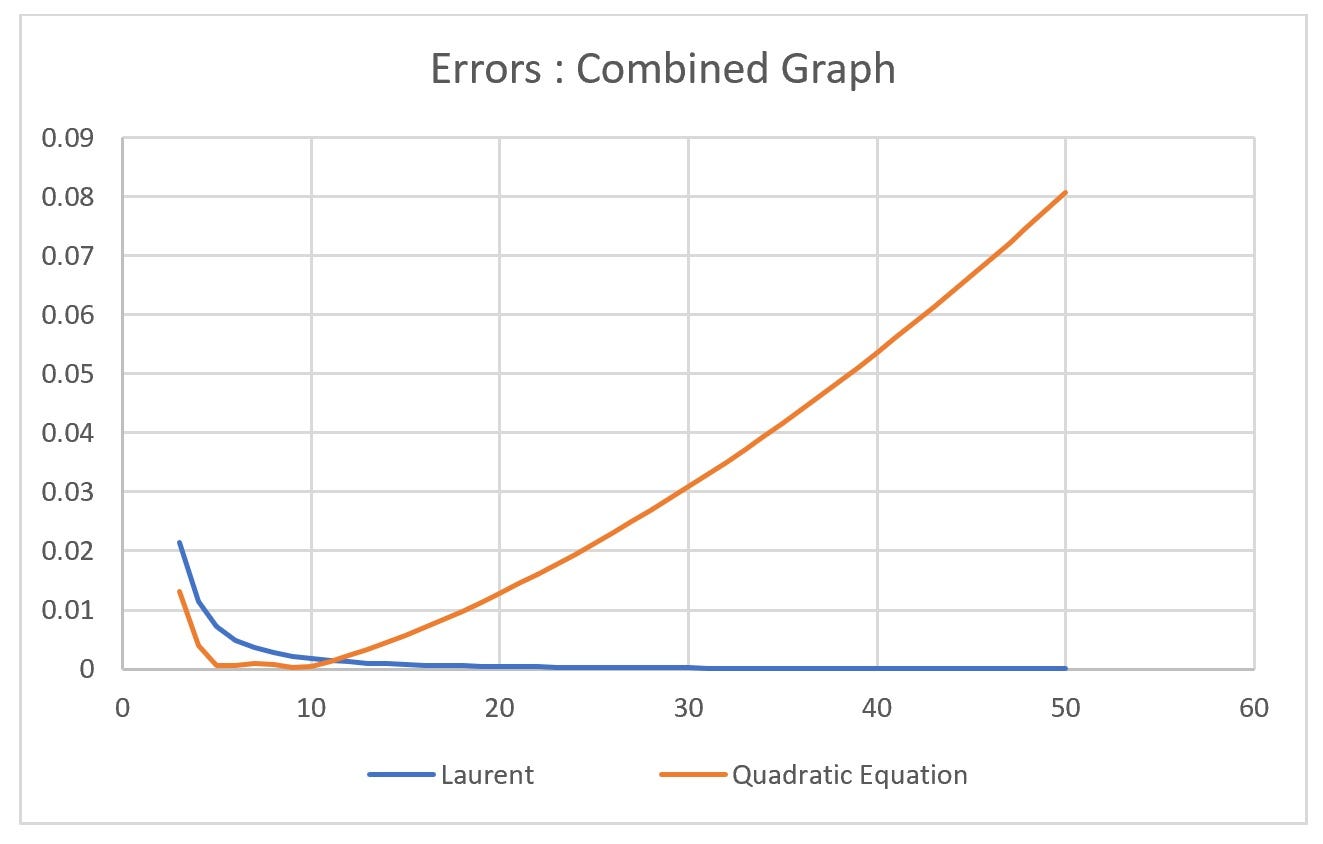
The absolute errors are still mighty small compared to the actual areas of each polygon, but they do seem to be growing as the number of sides increase. Sameer feels that his quadratic formula is will be a good service to high-school students who don’t yet know trigonometry but want a good way to approximate the areas of regular polygons.

I am impressed with Sameer’s play of mathematics — real and valid mathematics — and his sophistication of thought that comes from not trusting patterns but pushing ideas to use ideas inspired by patterns nonetheless to create powerful models. Playing through trial and error, guessing and checking, is mathematics!

Well done Sameer! You’ve exhibited the power and joy of independent creative and innovative mathematical thinking and doing!

Endnote:

For N large, a regular N-gon with side length 1, and hence perimeter N, is well approximated by a circle of perimeter N. The area of such a circle is

pi*(N/(2*pi))² = N² /4*pi.