STL原始碼學習----lower_bound和upper_bound演算法

ForwardIter lower_bound(ForwardIterfirst, ForwardIterlast,const _Tp& val)返回一個非遞減序列[first, last)中的第一個大於等於值val的位置。

 ForwardIter upper_bound(ForwardIter first, ForwardIterlast, const _Tp&val)返回一個非遞減序列[first, last)中第一個大於val的位置。

    lower_bound和upper_bound如下圖所示：

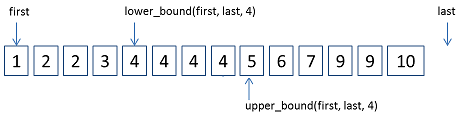
1， lower_bound

　　這個序列中可能會有很多重複的元素，也可能所有的元素都相同，為了充分考慮這種邊界條件，STL中的lower_bound演算法總體上是才用了二分查詢的方法，但是由於是查詢序列中的第一個出現的值大於等於val的位置，所以演算法要在二分查詢的基礎上做一些細微的改動。

     首先是我修改資料結構課本上的二分查詢實現的lower_bound演算法：

int my_lower_bound(int *array, int size, int key)
{
    int first = 0, last = size;
    int middle, pos=0;       //需要用pos記錄第一個大於等於key的元素位置
 


    while(first < last)
    {
        middle = (first+last)/2;
        if(array[middle] < key){      //若中位數的值小於key的值，我們要在右邊子序列中查詢，這時候pos可能是右邊子序列的第一個
            first = middle + 1;
            pos = first;
        }
        else{
            last = middle;           //若中位數的值大於等於key，我們要在左邊子序列查詢，但有可能middle處就是最終位置，所以我們不移動last,
 

            pos = last;              //而是讓first不斷逼近last。
        }
    }
    return pos;
}

　　STL中的實現比較精巧，下面貼出原始碼： 

//這個演算法中，first是最終要返回的位置
int lower_bound(int *array, int size, int key)
{
    int first = 0, middle;
    int half, len;
    len = size;

    while(len > 0) {
        half = len >> 1;
        middle = first + half;
        if(array[middle] < key) {     
            first = middle + 1;          
            len = len-half-1;       //在右邊子序列中查詢
        }
        else
            len = half;            //在左邊子序列（包含middle）中查詢
    }
    return first;
}

2， upper_bound

upper_bound返回的是最後一個大於等於val的位置，也是有一個新元素val進來時的插入位置。

我依然將二分查詢略做修改：

int my_upper_bound(int *array, int size, int key)
{
    int first = 0, last = size;
    int middle, pos = 0;

    while(first < last)
    {
        middle = (first+last)/2;
        if(array[middle] > key){     //當中位數大於key時，last不動，讓first不斷逼近last
            last = middle;
            pos = last;
        }
        else{
            first = middle + 1;     //當中位數小於等於key時，將first遞增，並記錄新的位置
            pos = first;
        }
    }
    return pos;
}

下面的程式碼是STL中的upper_bound實現：

int upper_bound(int *array, int size, int key)
{
    int first = 0, len = size-1;
    int half, middle;

    while(len > 0){
        half = len >> 1;
        middle = first + half;
        if(array[middle] > key)     //中位數大於key,在包含last的左半邊序列中查詢。
            len = half;
        else{
            first = middle + 1;    //中位數小於等於key,在右半邊序列中查詢。
            len = len - half - 1;
        }
    }
    return first;
}

lower_bound和upper_bound用法示例

#include <iostream>
#include <algorithm>

using namespace std;
int a[15];
int main() {
    int t;
    cin>>t;
    while (t--) {
        int n;
        scanf("%d", &n);
        for (int i = 0; i < n; i++){
            cin>>a[i];}
        int kk = lower_bound(a, a + n + 1, 2)-a; //序列的長度是n+1，在[a,a+n+1)中找出大於等於2的位置，這個位置是從0開始的。
        cout<<"未排序序列中 大於等於2的位置："<<kk+1<<endl;

        sort(a, a + n);    //第一個
        for (int i = 0; i < n; i++){
            cout<<a[i]<<" ";}
            cout<<endl;
        int kk2 = lower_bound(a, a + n + 1, 2)-a; //序列的長度是n+1，在[a,a+n+1)中找出大於等於2的位置，這個位置是從0開始的。
        cout<<"排好序序列中 大於等於2的位置："<<kk2+1<<endl;
        return 0;
    }
}