# 背景 考試想造浮點數然後發現不會 正好下午被虎哥茶話會 談到了一些不會的問題balabala的 被告知$C++11$有些神奇特性（哦豁） 然後就學習了一手看上去沒什麼用的隨機數生成器$QwQ$ # 函式 ## random_device 標準庫提供了一個非確定性隨機數生成裝置 在$Linux$的實現中,是讀取$/dev/urandom$裝置 random_device提供()操作符,用來返回一個min()到max()之間的一個數字 如果是$Linux(Unix Like或者Unix)$下,都可以使用這個來產生高質量的隨機數,可以理解為**真隨機數** （以上都是廢話，其實和最原始的c++的rand()用法一樣，不過**真隨機數**好評） ``` #include #include using namespace std; signed main(){ random_device rand; cout << rand() << endl; return 0; } ``` ## default_random_engine 一個隨機化的前置引擎 給後面要用到的函式生成一個隨機節點（時間戳balabala隨便理解一下就好，並沒有什麼卵用，就是讓後面的函式隨機化更強） 和上面提到的random_device不同的是，這個需要提供時間種子，看上去和rand也沒什麼區別。。。 ```cpp #include #include using namespace std; signed main(){ default_random_engine rand(time(NULL)); cout << rand() << endl; return 0; } ``` ## uniform_int_distribution 好了乾貨來了 該函式的作用是生成一個[a,b]範圍內的整數 定義的時候傳進去相應的引數（資料範圍即可） ```cpp uniform_int_distribution rand1(-100, 100); ``` 呼叫的時候給時間種子（就是上面device寫的rand函式） ```cpp cout << rand1(rand) << " "; ``` ## uniform_real_distribution 最有用的東西還是這個實數域的隨機生成器 用法和上述int一樣 ```cpp uniform_real_distribution rand2(0.0, 1.0); cout << rand2(rand) << endl; ``` # 正態分佈normal_distribution 再來說一個常用的 正態分佈 正態分佈$N(μ,σ^2)$呈現經典的”鐘形曲線”的形狀，其中中心峰的$x$座標由$μ$給出，峰的寬度受$σ$控制。 正態分佈由兩個引數控制，$μ∈R$和$σ∈(0,∞)$ 分佈的標準差用$σ$表示，方差用$σ^2$表示 使用方法，第一個引數是$μ$，第二個是$σ$ ```cpp normal_distribution N(10.0, 5.0); ``` 為了方便直觀的看出資料分佈，把每次生成的資料出現次數+1，測試的時候輸出了資料分佈圖像 ```cpp for(register int i = 0; i < 10000; i++){ double num = nor(rand); if ((num >= 0.0) && (num < 20.0)) ++p[int(num)]; } for (int i = 0; i < 20; ++i) { cout << i << "-" << (i + 1) << ": "; cout << string(p[i] * 100 / 10000, '*') << endl; } ```  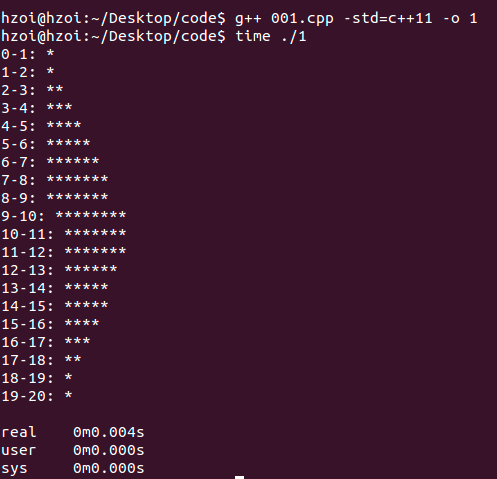具體要求按照具體題目要求，修改引數即可 # Code 最後把程式碼貼上一下，有需要自取就好 ```cpp #include #include using namespace std; int p[1000]; signed main(){ default_random_engine rand(time(NULL)); uniform_int_distribution rand1(-100, 100); uniform_real_distribution rand2(0.0, 1.0); cout << rand() << " "; cout << rand1(rand) << " "; cout << rand2(rand) << endl; normal_distribution nor(10.0, 5.0); for(register int i = 0; i < 10000; i++){ double num = nor(rand); if ((num >= 0.0) && (num < 20.0)) ++p[int(num)]; } for (int i = 0; i < 20; ++i) { cout << i << "-" << (i + 1) << ": "; cout << std::string(p[i] * 100 / 10000, '*') << std::endl; } return 0; } ``` # 小結 目前常用的這些 如果後續再有需求再補充吧 哦對了 編譯命令 ```cpp g++ 001.cpp -std=c++11 -o 1 ```