第n個陣元的訊號複數加權權值為an。

The signal in branch n is weighted with thecomplex weight an .

若參考陣元接收到的電場強度為E0exp(jΩt)，那麼整個陣列接收的總電壓E為

For an incoming electric field E0exp(jΩt)at the reference element, the total output voltage E can easily be shown to be(Stutzman and Thiele, 1998; Skolnik, 2001)

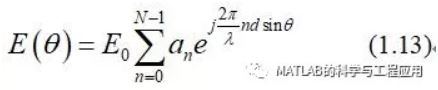
式（1.13）與加權序列{an}的離散傅立葉變換DFT形式是相似的。

This is similar in form to the discreteFourier transform (DFT) of the weight sequence {an}.

如同孔徑天線一樣，線性陣列的天線方向圖與傅立葉變換有關，這裡的加權序列（加權決定了電流分佈的特性）也是如此。

Like the aperture antenna, the antennapattern of the linear array thus involves a Fourier transform, this time of theweight sequence (which determines the current distribution in the antenna).

當an= 1，天線方向圖為我們熟悉的"aliased sinc"函式，其幅度為：

For the case where all the an =1, the pattern is the familiar “aliased sinc” function, whosemagnitude is

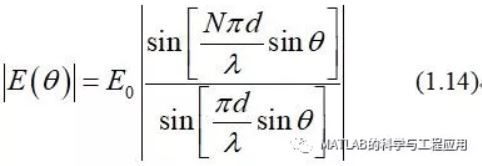
該函式與式（1.8）和圖1.6非常相似。

This function is very similar to that ofEq. (1.8) and Fig. 1.6.

如果天線陣元的數量N非常大（9個或更多），則總的孔徑大小D = Nd，3-dB波束寬度為0.89λ/D，第一旁瓣低於主瓣峰值13.2dB（均勻加權條件下）。

If the number of elements N is reasonablylarge (nine or more) and the product Nd is considered to be the total aperturesize D, the 3-dB beamwidth is 0.89λ/D, and the first sidelobe is 13.2 dB belowthe mainlobe peak; both numbers are the same as those of the uniformlyilluminated aperture antenna.

當然，通過改變幅度加權an，可以降低旁瓣電平，但也會帶來主瓣寬度的展寬。

Of course, by varying the amplitudes of theweights an , it is possible to reduce the sidelobes at the expenseof a broader mainlobe.

相位中心位於陣列的中心。

The phase center is at the center of thearray.

而真實的天線陣元通常並不是各向同性的全向均勻的輻射器。

Actual array elements are not isotropicradiators.

典型的天線陣元方向圖簡化模型通常使用一階近似，即：

A simple model often used as a first-orderapproximation to a typical element pattern Eel(θ) is

式（1.13）的右側被稱為陣列因子AF(θ)，則複合的陣列方向圖表達式為：

The right-hand side of Eq. (1.13) is thencalled the array factor AF(θ), and the composite radiation pattern becomes

由於餘弦函式隨θ變化緩慢，所以尤其在θ = 0°附近考慮陣元方向圖的複合陣列方向圖的變化並不明顯，因此，波束寬度和第一副瓣電平並不會發生很大的變化。

Because the cosine function is slowlyvarying in θ, the beamwidth and first sidelobe level are not greatly changed byincluding the element pattern for signals arriving at angles near broadside(near θ = 0°).

陣元方向圖確實降低了遠區旁瓣，從而降低了陣列對遠離正側向入射訊號的靈敏度。

The element pattern does reduce distantsidelobes, thereby reducing sensitivity to waves impinging on the array fromoff broadside.

到目前為止，我們是根據發射天線方向圖（對於孔徑天線）或接收方向圖（對於天線陣列）進行討論的，但不是針對兩者。

The discussion so far has been phrased interms of the transmit antenna pattern (for aperture antennas) or the receivepattern (for arrays), but not both.

天線方向圖都是指單程天線方向圖。

The patterns described have been one-wayantenna patterns.

互易性定理保證了接收天線方向圖與發射天線方向圖是相同的(Balanis，2005)。

The reciprocity theorem guarantees that thereceive antenna pattern is identical to the transmit antenna pattern (Balanis,2005).

因此，對於單基地雷達，雙程天線方向圖（功率或電壓）僅僅是相應的單程方向圖的平方。

Consequently, for a monostatic radar, thetwo-way antenna pattern (power or voltage) is just the square of thecorresponding oneway pattern.

此外，天線相位中心在發射和接收模式下都是相同的。

It also follows that the antenna phasecenter is the same in both transmit and receive modes.

——本文譯自Mark A. Richards所著的《Fundamentals of Radar Signal Processing（Second edition）》

更多精彩文章請關注微訊號：