Jzzhu and Sequences
Time Limit:1000MS Memory Limit:262144KB 64bit IO Format:%I64d & %I64u
Submit

Status
Description
Jzzhu has invented a kind of sequences, they meet the following property:

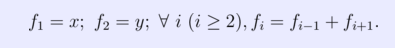
You are given x and y, please calculate fn modulo 1000000007(109 + 7).

Input
The first line contains two integers x and y(|x|, |y| ≤ 109). The second line contains a single integer n(1 ≤ n ≤ 2·109).

Output
Output a single integer representing fn modulo 1000000007(109 + 7).

Sample Input
Input
2 3
3
Output
1
Input
0 -1
2
Output
1000000006
Hint
In the first sample, f2 = f1 + f3, 3 = 2 + f3, f3 = 1.

In the second sample, f2 =  - 1;  - 1 modulo (109 + 7) equals (109 + 6).

構造矩陣之後套模板即可；

#include <stdio.h>
 

#include <iostream>
#include <string.h>
#include <algorithm>
#include <math.h>
#include <ctype.h>
#include <time.h>
#include <queue>

using namespace std;

const long long MOD = 1000000007;

struct node
{
    long long m[2][2];
}ans,base;
long long a,b;
int n;

node multi(node a,node b)
{
    node tmp;
    for
 
(int i=0;i<2;i++)
        for(int j=0;j<2;j++)
    {
        tmp.m[i][j] = 0;
        for(int k=0;k<2;k++)
        {
            tmp.m[i][j] = ((tmp.m[i][j] + a.m[i][k] * b.m[k][j]) % MOD + MOD)%MOD;
        }
    }
    return tmp;
}

void fast_mod(int n)// 求矩陣 base 的  n 次冪
{
    base.m[0][0] = 0;base.m[0][1] = 1;
    base.m[1][0] = -1;base.m[1][1] = 1;
    ans.m[0][0] = ans.m[1][1] = 1;// ans 初始化為單位矩陣
    ans.m[0][1] = ans.m[1][0] = 0;
    while (n)
    {
        if (n&1) //實現 ans *= t; 其中要先把 ans賦值給 tmp，然後用 ans = tmp * t
            ans = multi(ans,base);
        base = multi(base,base);
        n>>=1;
    }
}

int main()
{
    while (scanf("%lld %lld %d",&a,&b,&n)!=EOF)
    {
        if (n==1)
        {
            printf("%lld\n",(a%MOD + MOD)%MOD);
            continue;
        }
        if (n==2)
        {
            printf("%lld\n",(b%MOD + MOD)%MOD);
            continue;
        }
        fast_mod(n-2);
        long long anss = (((ans.m[1][0]*a+ans.m[1][1]*b) % MOD) + MOD) % MOD;
        printf("%lld\n",anss);
    }
    return 0;
}