題目大意

給出一個數N代表有多少個矩形，然後下面有N個數代表每個矩形的高度，寬度均為1。最後求這N個矩形組成的最大面積！

解題思路

很容易想到的就是使用單調棧。維護一個從棧頂到棧低減小的棧！
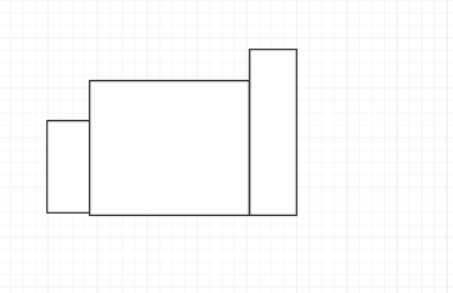
每次遇到棧頂的元素大於要壓入的元素就要，出棧然後和當前的最大面積取最大值，這樣一直下去，最後棧中就剩下一個從棧頂到棧低減小的棧。最後變為的棧就像下面這個圖一樣！

AC程式碼

我看的poj的discuss裡面不讓用long long和lld，事實證明是錯。我就是用這個過的！

#include<cstdio>
#include<stack>
#include<algorithm>
 

#include<iostream>
using namespace std;
typedef long long LL;
typedef pair<LL,LL> PII;
const int MX = 100000 + 5;

int main()
{
    int N;
    int height;
    while(~scanf("%d",&N) &&N)
    {
        stack<PII>Q;
        LL ans = 0;
        for(int i=0;i<N;i++)
        {
            scanf
 
("%d",&height);
            LL width = 0;
            while(!Q.empty() && Q.top().first>=height ){ //當棧頂元素大於當前元素
                LL tmpH = Q.top().first;
                LL tmpW = Q.top().second;
                Q.pop();width+=tmpW;//每次出棧的時候，就是計算以這個矩形的高度為最長高度的最大面積,
                //因為左邊的比他小，所以只需要向右延伸就可以了
 

                //所以每次出棧的時候，就是出棧後的棧頂元素寬度+1
                ans = max(ans,tmpH*width);//如果大於當前的最大面積就更新
            }
            Q.push(make_pair((LL)height,(LL)width+1));
        }
        int tmp = 0;
        while(!Q.empty()){
            ans = max(ans,Q.top().first*(tmp+Q.top().second));//原理和上面一樣
            tmp+=Q.top().second;Q.pop();
        }
        printf("%lld\n",ans);
    }
    return 0;
}