題目：

You have a list of points in the plane. Return the area of the largest triangle that can be formed by any 3 of the points.

Example:
Input: points = [[0,0],[0,1],[1,0],[0,2],[2,0]]
Output: 2
Explanation: 
The five points are show in the figure below. The red triangle is the largest.

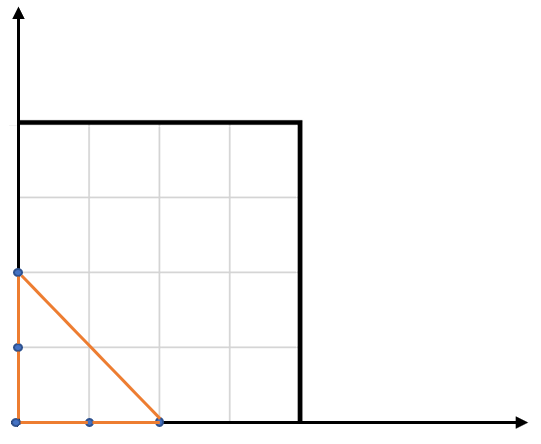
Notes:

• 3 <= points.length <= 50
  .
• No points will be duplicated.
•  -50 <= points[i][j] <= 50.
• Answers within 10^-6 of the true value will be accepted as correct.

思路：

我剛剛開始的時候覺得可能有比暴力法更好的演算法，後來還是沒發現，就只好用暴力法了。演算法的時間複雜度是O(n^3)，其中n是points中頂點的個數。

還有一種方法，就是我們知道面積最大的三角形的三個頂點一定是位於points的凸包上的。所以可以首先用O(nlogn)的時間複雜度求出points的凸包，然後再對凸包上的頂點採用暴力搜尋，這樣時間複雜度就降為O(m^3)，其中m是points的凸包上的頂點的個數。然而在最壞情況下O(m) == O(n)，所以演算法的時間複雜度並沒有實質的改進。

程式碼：

class Solution {
public:
    double largestTriangleArea(vector<vector<int>>& points) {
        double max_area = 0.0, area = 0.0;
        for (auto &p1 : points) {
            for (auto &p2 : points) {
                for (auto &p3 : points) {
                    area = abs(p1[0] * p2[1] + p2[0] * p3[1] + p3[0] * p1[1] - 
                               p2[0] * p1[1] - p3[0] * p2[1] - p1[0] * p3[1]) / 2.0;
                    max_area = max(max_area, area);
                }
            }
        }
        return max_area;
    }
};