http://www.51nod.com/onlineJudge/questionCode.html#!problemId=1463

題意：

思路：

好題！

先對所有查詢進行離線處理，按照右區間排序，因為k一共最多只有10個，所有在該區間內的B數組，每次枚舉K值，通過這樣的方式來得到另外一個B值。但是這樣得到的B值它在B數組中的位置必須在當前數的左邊。如下圖：（j為當前數在B數組中的位置，pos為計算得到的另一個B值在數組中的位置）

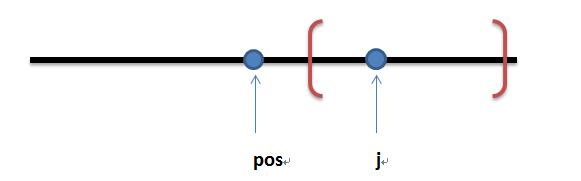
這兩個數的和記錄在pos中，這裏pos的位置必須在j的左邊，假設第q個查詢區間如上圖所示（請在腦中將pos和j互換一下），那麽此時j就沒包含在該區間內，這樣一來，查詢得到的值就會有錯。因為我們是按照右區間排序的，所以右區間會不斷擴大，只要左邊被覆蓋的，那麽右邊的數肯定是在該區間內的。

用線段樹維護即可。具體請參見代碼。

  1 #include<iostream>
  2 #include<algorithm>
  3 #include<cstring>
  4 #include<cstdio>
  5 #include<vector>
  6 #include<stack>
  7 #include<queue>
  8 #include<cmath>
  9 #include<map>
 10 #include<set>
 11
 
 using namespace std;
 12 typedef long long ll;
 13 const int INF = 0x3f3f3f3f;
 14 const int maxn=1e5+5;
 15 
 16 int n,q,m;
 17 int A[maxn],B[maxn],K[15],B_pos[maxn],ans[maxn];
 18 
 19 struct node
 20 {
 21     int l,r,id;
 22     bool operator<(const node& rhs) const
 23     {
 
 24         return r<rhs.r;
 25     }
 26 }Q[maxn];
 27 
 28 int MAX[maxn<<2];
 29 int val[maxn<<2];
 30 
 31 void build(int l, int r, int o)
 32 {
 33     val[o]=0;
 34     if(l==r)  return;
 35     int mid=(l+r)>>1;
 36     build(l,mid,o<<1);
 37     build(mid+1,r,o<<1|1);
 38 }
 39 
 40 void update(int l, int r, int pos, int x, int o)
 41 {
 42     val[o]=max(val[o],x);
 43     if(l==pos && r==pos)  return;
 44     int mid=(l+r)>>1;
 45     if(pos<=mid)  update(l,mid,pos,x,o<<1);
 46     else   update(mid+1,r,pos,x,o<<1|1);
 47 }
 48 
 49 int query(int ql, int qr, int l, int r, int o)
 50 {
 51     if(ql<=l && qr>=r)  return val[o];
 52     int mid=(l+r)>>1;
 53     int res=0;
 54     if(ql<=mid)  res=max(res,query(ql,qr,l,mid,o<<1));
 55     if(qr>mid)   res=max(res,query(ql,qr,mid+1,r,o<<1|1));
 56     return res;
 57 }
 58 
 59 int main()
 60 {
 61    //freopen("in.txt","r",stdin);
 62     scanf("%d%d%d",&n,&q,&m);
 63     for(int i=1;i<=n;i++)  scanf("%d",&A[i]);
 64     for(int i=1;i<=n;i++)  {scanf("%d",&B[i]);B_pos[B[i]]=i;}
 65     for(int i=1;i<=m;i++)  scanf("%d",&K[i]);
 66     for(int i=1;i<=q;i++)
 67     {
 68         scanf("%d%d",&Q[i].l,&Q[i].r);
 69         Q[i].id=i;
 70     }
 71     sort(Q+1,Q+q+1);
 72     build(1,n,1);
 73     int s=1;
 74     memset(MAX,0,sizeof(MAX));
 75     for(int i=1;i<=q;i++)
 76     {
 77         int r=Q[i].r;
 78         for(int j=s;j<=r;j++)
 79         {
 80             for(int k=1;k<=m;k++)
 81             {
 82                 int tmp_B=B[j]+K[k];
 83                 int pos=B_pos[tmp_B];  //得到tmp_B在B數組中的位置
 84                 if(tmp_B<=n && pos<j && A[pos]+A[j]>MAX[pos])
 85                 {
 86                     MAX[pos]=A[pos]+A[j];  //此時pos位置的最大值是由pos和[1,j)之間的一個數相加而成
 87                     update(1,n,pos,MAX[pos],1); //更新線段樹
 88                 }
 89                 tmp_B=B[j]-K[k];
 90                 pos=B_pos[tmp_B];
 91                 if(tmp_B>=1 && pos<j && A[pos]+A[j]>MAX[pos])
 92                 {
 93                     MAX[pos]=A[pos]+A[j];
 94                     update(1,n,pos,MAX[pos],1);
 95                 }
 96             }
 97         }
 98         ans[Q[i].id]=query(Q[i].l,Q[i].r,1,n,1); //查詢該區間內的最大值
 99         s=r;
100     }
101     for(int i=1;i<=q;i++)  printf("%d\n",ans[i]);
102     return 0;
103 }

51nod 1463 找朋友（線段樹+離線處理）