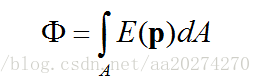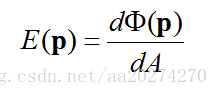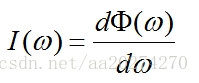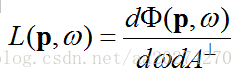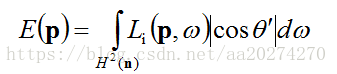1. Light and Color

The wavelength, λ, of light determines its “color”

2. Describe light by a spectrum (光譜)

“Intensity” of light at each wavelength

A graph of “intensity” vs. wavelength

We care about wavelengths in the visible spectrum: between the infra-red (700nm) and the ultra-violet (400nm)

3.  Examples

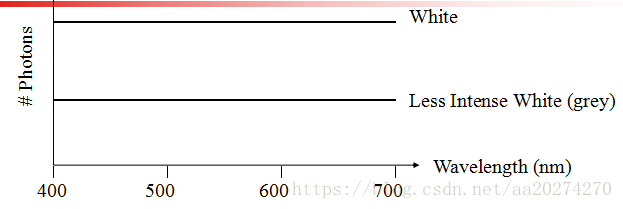
White and Grey

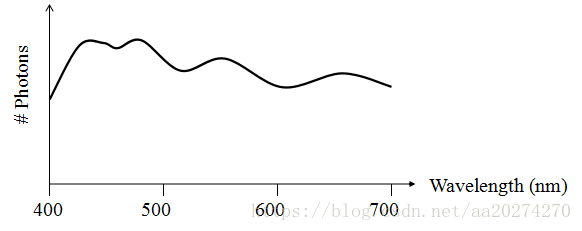
Helium Neon Laser(鐳射)

Normal Daylight

Tungsten Lightbulb

4.  個人理解：

一條光線 可以用 上面的 SPD 圖表來表示，SPD圖表描述了 光線的每一個波長上光子數目的 情況，SPD圖表可以轉換為 XYZ，XYZ可以轉為了RGB，所以，就有了一條光線對應視覺中的什麼顏色。

5. Radiometric Quantities

a. Flux: Total in some domain

b. Irradiance: Per unit area

c. Intensity: Per unit angle

d. Radiance: Per unit angle per unit projected area

Basically, all the latter are differential(微分) quantities that have no meaning(沒有意義) until you integrate(積分) them over some domain Like you have to integrate speed to get distance traveled

5.1 Radiant Flux

a. Total amount of energy passing through a surface or region of space per unit time

Typically denoted by 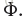 (Phi)

Also called power

Measured in watts (W) or joules/second (J/s)

b. Typically used for a light’s total output

You talk about a 60W light bulb

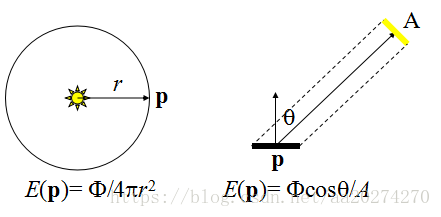
5.2 Irradiance

Irradiance is the power arriving at a surface, per unit area on the surface

Denoted E in PBR, or sometimes Ir or sometimes I

Units: Wm-2

5.3 Irradiance to Flux

Integrate over area:

Hence, we can also say (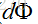 is flux density, varies over area):

5.4 Measuring Angle

The solid angle subtended by an object from a point P is the area of the projection of the object onto the unit sphere centered at P

Measured in steradians, sr

Definition is analogous to projected angle in 2D

If I’m at P, and I look out, solid angle tells me how much of my view is filled with an object

5.5 Intensity

Flux density per unit solid angle:

Only meaningful for point light sources (otherwise we would need some concept of the area of the light)

5.6 Radiance

Flux density per unit area perpendicular to the direction of travel, per unit solid angle:

Units Wm-2sr-1, power per unit area per unit solid angle

Radiance is the fundamental measurement

All others can be computed from it via integrals over area and/or directions

Radiance is constant along lines

No r2 falloff, the per unit solid angle takes care of it

5.7 Irradiance from Radiance

Integrate radiance over directions in the upper hemisphere:

cos term deals with projected solid angle. θ is angle between w and n (the normal)