LeetCode-Algorithms #005 Longest Palindromic Substring

英語學習時間
palindromic: [醫] 復發的, 再發的

在數學和計算機上,就指回文

這道題目就是找出給定字串中最長的迴文子串, 可以假定原字串的長度不超過1000

直接遍歷來做肯定是不難, 但也可以想見一定是慢得可以.

那麼我的另一個想法是, 從原串中的每一個字元, 或每兩個字元中間的空隙開始, 向左右兩邊判斷是否為迴文串, 最後找出最長的

 1 class Solution {
 2     public String longestPalindrome(String s) {
 3
 
         //首先排除特殊情況
 4         if (s == null || "".equals(s) || s.length() == 1) {
 5             return s;
 6         } else {
 7             //先建立一個空串以儲存結果
 8             String result = "";
 9             //獲取原字串的長度
10             int len = s.length();
11             
12             //因為之前已經排除了特殊情況, 所以到這裡原串的長度至少為2, 那麼為了找到最長的迴文子串, 我們只需要遍歷到倒數第二個字元即可
 

13             for (int i = 0; i < len - 1; i++) {
14                 //首先考慮以第i個字元為中心, 向兩側做判斷的情況
15                 int begin = i, end = i;
16                 while (true) {
17                     //如果begin或end的外側未達到邊界則繼續判斷
18                     if (begin > 0 && end < len - 1) {
19                         //
 
如果begin左側的字元和end右側的字元相同則向兩側延伸一位
20                         if (s.charAt(begin - 1) == s.charAt(end + 1)) {
21                             begin--;
22                             end++;
23                         } else { //反之則結束迴圈
24                             break;
25                         }
26                     } else { //如果begin或end已達到邊界則終止迴圈
27                         break;
28                     }
29                 }
30                 //判斷最後取得的迴文子串是否比之前儲存的結果長
31                 if (result.length() < (end - begin + 1)) {
32                     //如果是則替換結果
33                     result = s.substring(begin, end + 1);
34                 }
35 
36                 //再以i和i+1之間的空隙為中心向兩側進行判斷
37                 begin = i;
38                 end = i + 1;
39                 //如果i處的字元和i+1處不同則直接跳過, 反之則繼續進行判斷
40                 if (s.charAt(begin) == s.charAt(end)) {
41                     //後面內容同上半部分
42                     while (true) {
43                         if (begin > 0 && end < len - 1) {
44                             if (s.charAt(begin - 1) == s.charAt(end + 1)) {
45                                 begin--;
46                                 end++;
47                             } else {
48                                 break;
49                             }
50                         } else {
51                             break;
52                         }
53                     }
54                     if (result.length() < (end - begin + 1)) {
55                         result = s.substring(begin, end + 1);
56                     }
57                 }
58             }
59             //最後返回結果
60             return result;
61         }
62     }
63 }

 順利通過, 但是成績很一般, 作為一道經典題目, 強者們的速度可以說相當喪心病狂了,

這裡面涉及到一個經典演算法--Manacher's Algorithm, 也就是傳說中的馬拉車演算法, 今天研究了一下, 可以稍微談一談,

我上面的這種寫法, 時間複雜度是O(n2), 沒有進行任何的優化, 如果跳過一些不必要的計算, 應該還可以快上不少, 但馬拉車可以做到O(n)的時間複雜度, 相當高端了

具體實現的思路是這樣的:

在我上面的寫法中, 從字串的第一個字元開始進行遍歷時, 每次檢驗都要分兩種情況:

1) 以該字元為中心向兩側檢驗

2) 以該字元與該字元的下一個字元間的空隙為中心向兩側檢驗

而在馬拉車演算法中, 會首先向字串的各個字元間插入一個特殊字元(多用#)

這樣 babad 就會變成 #b#a#b#a#d#, aa 就會變成 #a#a#, 這樣就避免了分類討論的情形.

之後, 我們建立一個與新字串長度相同的整數陣列, 每個位置儲存以該位置為中心的迴文子串的長度,如:

#b#a#b#a#d#
13171713131

為了方便計算, 我們再將這個結果長度都除以二:

#b#a#b#a#d#
01030301010

如果有了這個陣列, 那麼最長的迴文子串顯然也就找到了, 我們的目標就是計算出這個陣列, 讓我們看一下馬拉車的思路

#a#b#a#b#a#b#a#b#a#c#d#e#

如對上面這個陣列進行遍歷, 

第一位#對應的顯然是0, 第二位a對應的是1, 第三位#是0, 第四位b是3,第五位#是0,第六位a是5,第7位#是0,

對第8位的b進行判斷時, 由於以第6位a為中心的迴文子串會向左右各覆蓋5位, 那麼顯然第8位的b在這個範圍之內.

那麼, 以第8位b為中心的迴文子串最少應該與以第四位b為中心的迴文子串等長, 也就是左右各覆蓋三位,

因此我們可以從第8位b的左右各第四位開始測試, 這樣就節約了計算量, 最後得到第8位b對應的數值是7.

按照這個思路, 我們可以在遍歷中設定一個最右邊界, 在對特定字元進行檢驗是, 如果該字元在已知的迴文子串覆蓋下, 

那麼就計算其相對最右邊界迴文串中心對稱的位置, 得出已知迴文串的長度.

判斷該長度和右邊界，如果達到了右邊界，那麼需要進行中心擴充套件探索。

當然，如果第3步該字元沒有在最右邊界的“羽翼”下，則直接進行中心擴充套件探索。進行中心擴充套件探索的時候，同時又更新右邊界.

上面的敘述部分參考(抄襲)了下面這篇文章.

這裡貼一個寫得很漂亮的答案, 雖然用的是馬拉車的思路, 但並不完全按照上面的步驟實現

 1 public class Solution {
 2     
 3     int len = 0, maxLength = 0, init = 0;
 4     
 5     public String longestPalindrome(String s) {
 6         
 7         char[] chars = s.toCharArray();
 8         
 9         len = s.length();
10         
11         if (len <= 1) return s;
12         
13         for (int i = 0; i < len; i++) {
14             i = manacher(chars, i);
15         }
16         return s.substring(init, init + maxLength);
17     }
18     
19     public int manacher(char[] chars, int k) {
20         
21         int i = k - 1, j = k;
22         while (j < len - 1 && chars[j] == chars[j + 1]) j++;
23         int nextCenter = j++;
24         
25         while (i >= 0 && j < len && chars[i] == chars[j]) {
26             i--;
27             j++;
28         }
29         
30         if (j - i - 1 > maxLength) {
31             maxLength = j - i - 1;
32             init = i + 1;
33         }
34         
35         return nextCenter;
36     }
37     
38 }

LeetCode-Database #180 Consecutive Numbers

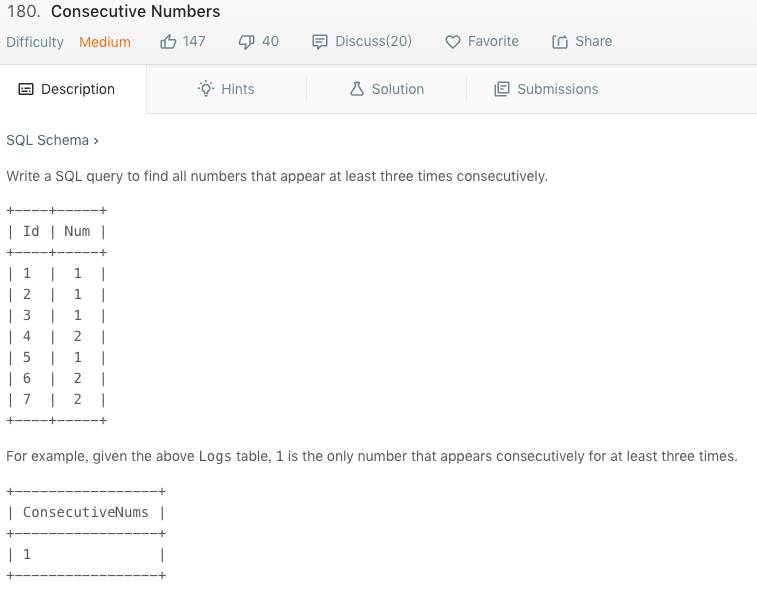
找出所有連續出現過至少三次的數字

 1 SELECT DISTINCT
 2     l1.Num AS ConsecutiveNums
 3 FROM
 4     Logs l1,
 5     Logs l2,
 6     Logs l3
 7 WHERE
 8     l1.Id = l2.Id - 1
 9     AND l2.Id = l3.Id - 1
10     AND l1.Num = l2.Num
11     AND l2.Num = l3.Num
12 ;

答案長這樣, 也沒什麼好說的了

再貼一個好一點的答案

 1 # Write your MySQL query statement below
 2 # select a.Num as ConsecutiveNums from Logs a inner join 
 3 # (select b.Id, b.Num from Logs b inner join Logs c
 4 # on b.Id=c.Id-1 and b.Num=c.Num) d
 5 # on a.Id=d.Id-1 and a.Num=d.Num
 6 # Group by a.Num
 7 
 8 SELECT DISTINCT(Num) AS ConsecutiveNums
 9 FROM (
10     SELECT
11     Num,
12     @counter := IF(@prev = Num, @counter + 1, 1) AS how_many_cnt_in_a_row,
13     @prev := Num
14     FROM Logs y, (SELECT @counter:=1, @prev:=NULL) vars
15 ) sq
16 WHERE how_many_cnt_in_a_row >= 3