Sum

Time Limit: 2000/1000 MS (Java/Others)    Memory Limit: 131072/131072 K (Java/Others)
Total Submission(s): 1320    Accepted Submission(s): 570


Problem Description
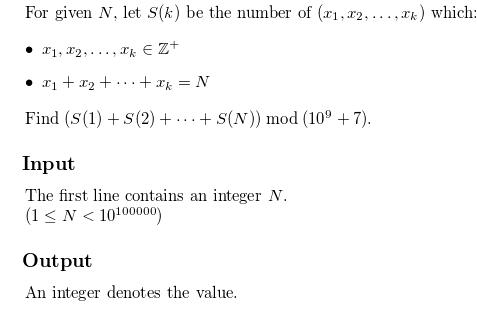
Sample Input 2
Sample Output 2 Hint 1. For N = 2, S(1) = S(2) = 1. 2. The input file consists of multiple test cases.
Source
Recommend zhuyuanchen520   |   We have carefully selected several similar problems for you:  

4955 4954 4953 4952 4951  思路：

一道整數劃分題目，不難推出公式：2^(n-1)，

根據費馬小定理：(2,MOD)互質，則2^(p-1)%p=1；

由於n非常大,所以這裡要用到費馬小定理:a^n%m ≡ (a^(n%(m-1)) * a^(m-1))%m=a^(n%(m-1)) %m

 ac程式碼

#include<stdio.h>
#include<math.h>
#include<string.h>
#define mod 1000000007
__int64 fun(__int64 a,__int64 n)
{
	__int64 s=1;
	while(n)
	{
		if(n%2)
			s=s*a%mod;
		a=a*a%mod;
		n/=2;
	}
	return s;
}
int main()
{
	char s[1000000];
	while(scanf("%s",s)!=EOF)
	{
		__int64 sum=0; int i,len=strlen(s);
		getchar();
		for(i=0;i<len;i++)
		{
			sum=(sum*10+s[i]-'0')%(mod-1);//注意這種對字串求餘的方式
		}
		printf("%I64d\n",fun(2,sum-1));
	}
}