poj1039 Pipe【計算幾何】
阿新 • • 發佈:2018-10-02
sid sha n) ria lan lag != sum mit 含【求直線交點】、【判斷直線與線段相交】模板
Pipe
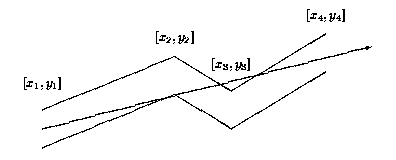
Each pipe component consists of many straight pipes connected tightly together. For the programming purposes, the company developed the description of each component as a sequence of points [x1; y1], [x2; y2], . . ., [xn; yn], where x1 < x2 < . . . xn . These are the upper points of the pipe contour. The bottom points of the pipe contour consist of points with y-coordinate decreased by 1. To each upper point [xi; yi] there is a corresponding bottom point [xi; (yi)-1] (see picture above). The company wants to find, for each pipe component, the point with maximal x-coordinate that the light will reach. The light is emitted by a segment source with endpoints [x1; (y1)-1] and [x1; y1] (endpoints are emitting light too). Assume that the light is not bent at the pipe bent points and the bent points do not stop the light beam.
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions:11940 | Accepted: 3730 |
Description
The GX Light Pipeline Company started to prepare bent pipes for the new transgalactic light pipeline. During the design phase of the new pipe shape the company ran into the problem of determining how far the light can reach inside each component of the pipe. Note that the material which the pipe is made from is not transparent and not light reflecting.
Each pipe component consists of many straight pipes connected tightly together. For the programming purposes, the company developed the description of each component as a sequence of points [x1; y1], [x2; y2], . . ., [xn; yn], where x1 < x2 < . . . xn . These are the upper points of the pipe contour. The bottom points of the pipe contour consist of points with y-coordinate decreased by 1. To each upper point [xi; yi] there is a corresponding bottom point [xi; (yi)-1] (see picture above). The company wants to find, for each pipe component, the point with maximal x-coordinate that the light will reach. The light is emitted by a segment source with endpoints [x1; (y1)-1] and [x1; y1] (endpoints are emitting light too). Assume that the light is not bent at the pipe bent points and the bent points do not stop the light beam.
Input
Output
The output file contains lines corresponding to blocks in input file. To each block in the input file there is one line in the output file. Each such line contains either a real value, written with precision of two decimal places, or the message Through all the pipe.. The real value is the desired maximal x-coordinate of the point where the light can reach from the source for corresponding pipe component. If this value equals to xn, then the message Through all the pipe. will appear in the output file.Sample Input
4 0 1 2 2 4 1 6 4 6 0 1 2 -0.6 5 -4.45 7 -5.57 12 -10.8 17 -16.55 0
Sample Output
4.67 Through all the pipe.
Source
Central Europe 1995
題意:
給n個點 構成兩條平行的折線
問從管道口出發的光線最遠能到達的橫坐標
思路:
最遠的光線一定是貼著管道的某兩個端點走的
現在枚舉這兩個端點 判斷其與後面折線的交點
剛開始沒想到判斷交點時 可以先判斷line 和 line(up[k], down[k])
這樣得到的k就是最小的不能達到的k
用這個k就可以拿來算line 和 line(up[k-1], up[k])以及line(down[k -1], down[k])的交點了
1 //#include <bits/stdc++.h> 2 #include<iostream> 3 #include<cmath> 4 #include<algorithm> 5 #include<stdio.h> 6 #include<cstring> 7 8 using namespace std; 9 typedef long long int LL; 10 11 #define zero(x) (((x) > 0? (x) : -(x)) < eps) 12 const double eps = 1e-8; 13 int sgn(double x) 14 { 15 if(fabs(x) < eps) return 0; 16 if(x < 0) return -1; 17 else return 1; 18 } 19 20 struct point{ 21 double x, y; 22 point(){} 23 point(double _x, double _y) 24 { 25 x = _x; 26 y = _y; 27 } 28 point operator -(const point &b)const 29 { 30 return point(x - b.x, y - b.y); 31 } 32 double operator ^(const point &b)const 33 { 34 return x * b.y - y * b.x; 35 } 36 double operator *(const point &b)const 37 { 38 return x * b.x + y * b.y; 39 } 40 void input() 41 { 42 scanf("%lf%lf", &x, &y); 43 } 44 }; 45 46 struct line{ 47 point s, e; 48 line(){} 49 line(point _s, point _e) 50 { 51 s = _s; 52 e = _e; 53 } 54 //0表示直線重合,1表示平行,2相交 55 pair<int, point>operator &(const line &b)const 56 { 57 point res = s; 58 if(sgn((s - e) ^ (b.s - b.e)) == 0){ 59 if(sgn((s - b.e) ^ (b.s - b.e)) == 0){ 60 return make_pair(0, res); 61 } 62 else return make_pair(1, res); 63 } 64 double t = ((s - b.s) ^ (b.s - b.e)) / ((s - e) ^ (b.s - b.e)); 65 res.x += (e.x - s.x) * t; 66 res.y += (e.y - s.y) * t; 67 return make_pair(2, res); 68 } 69 }; 70 71 //判斷直線與線段相交 72 bool seg_inter_line(line l1, line l2) 73 { 74 return sgn((l2.s - l1.e) ^ (l1.s - l1.e)) * sgn((l2.e - l1.e) ^ (l1.s - l1.e)) <= 0; 75 } 76 77 int n; 78 point up[100], down[100]; 79 int main() 80 { 81 while(scanf("%d", &n) != EOF && n != 0){ 82 for(int i = 0; i < n; i++){ 83 up[i].input(); 84 down[i].x = up[i].x; 85 down[i].y = up[i].y - 1.0; 86 } 87 88 89 bool flag = false; 90 double ans = -100000000.0; 91 int k; 92 for(int i = 0; i < n; i++){ 93 for(int j = i + 1; j < n; j++){ 94 for(k = 0; k < n; k++){ 95 if(seg_inter_line(line(up[i], down[j]), line(up[k], down[k])) == 0){ 96 break; 97 } 98 } 99 if(k >= n){ 100 flag = true; 101 break; 102 } 103 if(k > max(i, j)){ 104 if(seg_inter_line(line(up[i], down[j]), line(up[k - 1], up[k]))){ 105 pair<int, point>pr = line(up[i], down[j]) & line(up[k - 1], up[k]); 106 point p = pr.second; 107 ans = max(ans, p.x); 108 } 109 if(seg_inter_line(line(up[i], down[j]), line(down[k - 1], down[k]))){ 110 pair<int, point>pr = line(up[i], down[j]) & line(down[k - 1], down[k]); 111 point p = pr.second; 112 ans = max(ans, p.x); 113 } 114 } 115 116 for(k = 0; k < n; k++){ 117 if(seg_inter_line(line(down[i], up[j]), line(up[k], down[k])) == 0){ 118 break; 119 } 120 } 121 if(k >= n){ 122 flag = true; 123 break; 124 } 125 if(k > max(i, j)){ 126 if(seg_inter_line(line(down[i], up[j]), line(up[k - 1], up[k]))){ 127 pair<int, point>pr = line(down[i], up[j]) & line(up[k - 1], up[k]); 128 point p = pr.second; 129 ans = max(ans, p.x); 130 } 131 if(seg_inter_line(line(down[i], up[j]), line(down[k - 1], down[k]))){ 132 pair<int, point>pr = line(down[i], up[j]) & line(down[k - 1], down[k]); 133 point p = pr.second; 134 ans = max(ans, p.x); 135 } 136 } 137 } 138 if(flag){ 139 break; 140 } 141 } 142 //cout<<ans<<endl; 143 if(flag){ 144 printf("Through all the pipe.\n"); 145 } 146 else{ 147 printf("%.2f\n", ans); 148 } 149 } 150 return 0; 151 }
poj1039 Pipe【計算幾何】
