Description

Assume the coasting is an infinite straight line. Land is in one side of coasting, sea in the other. Each small island is a point locating in the sea side. And any radar installation, locating on the coasting, can only cover d distance, so an island in the sea can be covered by a radius installation, if the distance between them is at most d. 

We use Cartesian coordinate system, defining the coasting is the x-axis. The sea side is above x-axis, and the land side below. Given the position of each island in the sea, and given the distance of the coverage of the radar installation, your task is to write a program to find the minimal number of radar installations to cover all the islands. Note that the position of an island is represented by its x-y coordinates. 

 
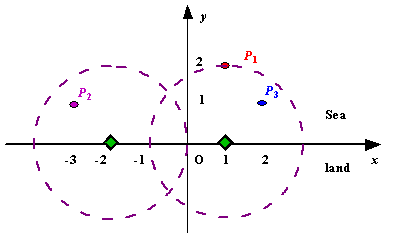
Figure A Sample Input of Radar Installations

Input

The input consists of several test cases. The first line of each case contains two integers n (1<=n<=1000) and d, where n is the number of islands in the sea and d is the distance of coverage of the radar installation. This is followed by n lines each containing two integers representing the coordinate of the position of each island. Then a blank line follows to separate the cases. 

The input is terminated by a line containing pair of zeros 

Output

For each test case output one line consisting of the test case number followed by the minimal number of radar installations needed. "-1" installation means no solution for that case.

Sample Input

3 2
1 2
-3 1
2 1

1 2
0 2

0 0

Sample Output

Case 1: 2
Case 2: 1

Source

這道題就是一道貪心題，思路並不難，但是悲催的是WA了5次，居然是因為如果不符合條件時輸出-1時直接輸出了-1，前面沒帶Case。。。。sad。。。害得我把點的排序按點排了一次，又按區間左端點排了一次。。不過事後發現這兩種排序都可以。。 本題貪心思路是把點轉化為在x軸座標上的區間（即能保證覆蓋該小島的雷達所有可能位置的集合），然後按點的順序排也行，按左端點排也行。然後最左邊的依次向右遍歷，如果下一個區間的最左端在上一個雷達的右端，顯然需要放一個新雷達；如果在左端的話，則需要判斷最右端了，如果最右端也在上個雷達左端的話，那麼這個雷達顯然不能覆蓋當前這個小島，需要把雷達位置調整為當前區間的最右端，這樣既能覆蓋之前的也能覆蓋現在的；如果最右端在上個雷達右端，則無需調整也無需放置新雷達。

#include <stdio.h>
#include <stdlib.h>
#include <string.h>
#include <math.h>
#include <algorithm>
using namespace std;
struct node
{
    double zuo, you;
} fei[2100], t;
int cmp(node a, node b)
{
    return a.zuo < b.zuo;
}
int x[2100],y[2100];
int main()
{
    int i, j, n, d, num, flag, s=0;
    double z, p;
    while(scanf("%d%d",&n,&d)!=EOF)
    {
        if(n==0&&d==0) break;
        s++;
        flag=0;
        for(i=0; i<n; i++)
        {
            scanf("%d%d",&x[i],&y[i]);
            if(y[i]>d)
                flag=1;
        }
        if(flag)
            printf("Case %d: -1\n",s);
        else
        {
            for(i=0; i<n; i++)
            {
                z=sqrt(d*d*1.0-y[i]*y[i]*1.0);
                fei[i].zuo=(double)x[i]-z;
                fei[i].you=(double)x[i]+z;
            }
            sort(fei,fei+n,cmp);
            num=0;
            p=-100000000;
            for(i=0; i<n; i++)
            {
                if(fei[i].zuo>p)
                {
                    num++;
                    p=fei[i].you;
                }
                else if(fei[i].you<p)
                {
                    p=fei[i].you;
                }
            }
            printf("Case %d: %d\n",s,num);
        }
    }
    return 0;
}