\(\color{#0066ff}{ 題目描述 }\)

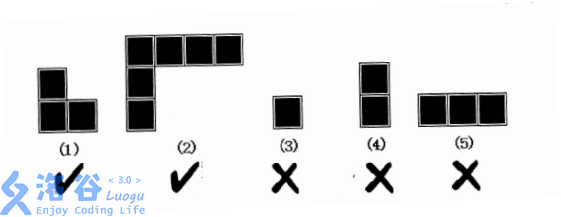
lxhgww的小名叫”小L“，這是因為他總是很喜歡L型的東西。小L家的客廳是一個R*C的矩形，現在他想用L型的地板來鋪滿整個客廳，客廳裡有些位置有柱子，不能鋪地板。現在小L想知道，用L型的地板鋪滿整個客廳有多少種不同的方案？需要注意的是，如下圖所示，L型地板的兩端長度可以任意變化，但不能長度為0。

鋪設完成後，客廳裡面所有沒有柱子的地方都必須鋪上地板，但同一個地方不能被鋪多次。

\(\color{#0066ff}{輸入格式}\)

輸入的第一行包含兩個整數，R和C，表示客廳的大小。接著是R行，每行C個字元。'_'表示對應的位置是空的，必須鋪地板；'*'表示對應的位置有柱子，不能鋪地板。

\(\color{#0066ff}{輸出格式}\)

輸出包含q行，第i行為m[i]個整數，該行的第j(j=1，2...，，m[i])個數表示第i年被授權的聚居地h[j]的臨時議事處管理的種族個數。

\(\color{#0066ff}{輸入樣例}\)

3 3
___
_*_
___

\(\color{#0066ff}{輸出樣例}\)

8

\(\color{#0066ff}{資料範圍與提示}\)

測試點編號      資料範圍             
1,2             R*C<=25
3-5             R*C<=100並且（R=2或者C=2）
6-10            R*C<=100
 

\(\color{#0066ff}{ 題解 }\)

一道不錯的插頭DP

情況要考慮全面

考慮插頭，0：無，1：未拐彎插頭，2：已拐彎插頭（題中要求必須是拐彎的磚）

套板子就行啦

再次宣告，我TM就不寫hash表，暴力卡常開\(O2\)，980ms卡過去！！！

// luogu-judger-enable-o2
#include<bits/stdc++.h>
#define LL long long
LL in() {
    char ch; LL x = 0, f = 1;
    while(!isdigit(ch = getchar()))(ch == '-') && (f = -f);
    for(x = ch ^ 48; isdigit(ch = getchar()); x = (x << 1) + (x << 3) + (ch ^ 48));
    return x * f;
}
std::unordered_map<int, int> f[2];
int n, m, s, t;
bool mp[120][120];
char getch() {
    char ch = getchar();
    while(ch != '*' && ch != '_') ch = getchar();
    return ch;
}
void init() {
    n = in(), m = in();
    static bool cp[120][120];
    for(int i = 1; i <= n; i++) 
        for(int j = 1; j <= m; j++)
            cp[i][j] = getch() == '_';
    if(m > n) {
        std::swap(n, m);
        for(int i = 1; i <= n; i++)
            for(int j = 1; j <= m; j++) {
                mp[i][j] = cp[j][i];
                if(mp[i][j]) s = i, t = j;
            }
    }
    else {
        for(int i = 1; i <= n; i++)
            for(int j = 1; j <= m; j++) {
                mp[i][j] = cp[i][j];
                if(mp[i][j]) s = i, t = j;
            }
    }
}
const int mod = 20110520;
int pos(int v, int x) { return (v << (x << 1LL)); }
void ins(int &x, int y) { x %= mod, (x += y) %= mod; }
int work() {
    int now = 0, nxt = 1;
    int ans = 0;
    f[now][0] = 1;
    int U = (1 << ((m + 1) << 1)) - 1;
    for(int i = 1; i <= n; i++) {
        for(int j = 1; j <= m; j++) {
            f[nxt].clear();
            for(auto &k:f[now]) {
                int S = k.first, val = k.second;
                int L = (S >> ((j - 1) << 1)) & 3, R = (S >> (j << 1)) & 3;
                if(!mp[i][j]) {
                    if(!L && !R) ins(f[nxt][S], val);
                    continue;
                }
                if(!L && !R) {
                    //right 1
                    if(mp[i][j + 1]) ins(f[nxt][S ^ pos(1, j)], val);
                    //down 1
                    if(mp[i + 1][j]) ins(f[nxt][S ^ pos(1, j - 1)], val);
                    //middle double 2
                    if(mp[i][j + 1] && mp[i + 1][j]) ins(f[nxt][S ^ pos(2, j - 1) ^ pos(2, j)], val);
                }
                //要麼直走還是1，要麼拐彎變成2
                else if(L == 0 && R == 1) {
                    if(mp[i + 1][j]) ins(f[nxt][S ^ pos(1, j - 1) ^ pos(1, j)], val);
                    if(mp[i][j + 1]) ins(f[nxt][S ^ pos(1, j) ^ pos(2, j)], val);
                }
                //要麼直走還是2，要麼停下變成0
                else if(L == 0 && R == 2) {
                    if(mp[i + 1][j]) ins(f[nxt][S ^ pos(2, j - 1) ^ pos(2, j)], val);
                    ins(f[nxt][S ^ pos(2, j)], val);
                }
                 //跟上面差不多
                else if(L == 1 && R == 0) {
                    if(mp[i][j + 1]) ins(f[nxt][S ^ pos(1, j - 1) ^ pos(1, j)], val);
                    if(mp[i + 1][j]) ins(f[nxt][S ^ pos(1, j - 1) ^ pos(2, j - 1)], val);
                }
                else if(L == 2 && R == 0) {
                    if(mp[i][j + 1]) ins(f[nxt][S ^ pos(2, j - 1) ^ pos(2, j)], val);
                    ins(f[nxt][S ^ pos(2, j - 1)], val);
                }
                 //必須拼上，沒有其他情況
                else if(L == 1 && R == 1) {
                    ins(f[nxt][S ^ pos(1, j - 1) ^ pos(1, j)], val);
                }
                 //這些情況都是不成立的
                else if(L == 1 && R == 2) {}
                else if(L == 2 && R == 1) {}
                else if(L == 2 && R == 2) {}
                if(i == s && j == t) {
                      //1 1 // 0 2 // 2 0 第一個是接上，後兩個是直接停下，都可以收集ans  
                    if(L + R == 2) ins(ans, val);
                }
            }
            std::swap(now, nxt);
        }
        f[nxt].clear();
        for(auto &k:f[now]) ins(f[nxt][(k.first << 2) & U], k.second);
        std::swap(nxt, now);
    }
    return ans;
}
int main() {
    init();
    printf("%d\n", work() % mod);
    return 0;
}